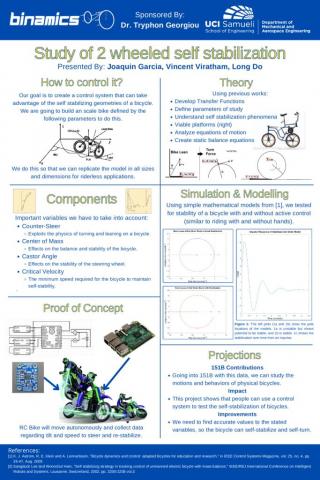
Self-stabilization Geometries for Two Wheeled Locomotion

Background:

Bicycles have been a popular method of transportation for over 200 years. Many different iterations of the bicycle have emerged as different needs arise: geared bikes, faster bikes, lighter bikes. Despite all the engineering involved in their evolution over time, there is still no common understanding for the stability of a bicycle. Unlike many other moving vehicles, such as cars, a bicycle only has two wheels, meaning it could easily tip over onto its side and fall. However, there is a phenomenon where a bicycle is able to self-stabilize and stay upright even without a rider, so long as it maintains a sufficiently large forward velocity. 

There are many theories regarding this phenomenon, and much discourse as to what actually causes it. Some have attributed bicycle stability to the gyroscopic effect of the front wheel. However, the gyroscopic effect can be canceled out by coupling a counter-rotating wheel to both the front and back wheel of a bicycle, and there will still be stability. Another theory involves the distance between the point of contact of the front wheel and the point where the steering axis intersects the ground. If the steering axis intersects the ground in front of the point of contact of the front wheel, then the front wheel may be able to self-align itself to the direction of motion similar to how a caster wheel on a shopping cart will align itself. However, previous literature has also experimented with a negative caster and once again maintained stability. The general idea regarding bicycle self-stability is that, during motion, as a bicycle begins to fall, the steering wheel will turn into the fall causing the bicycle to erect itself and stay standing.

Our team has explored mathematical models and experimented with physical models to determine what are the most important factors regarding bicycle stability and how we can use these ideas to create a self-stabilizing rider-less bicycle.

Goal and Objectives:

The purpose of this project is to create a control system that can utilize a self-stabilization two-wheel locomotion, so we can study and understand its behavior and equations of motion. To achieve this, sensors were placed on the two-wheel locomotion to collect data for the determination of the parameters that affect the stability through simulation on MATLAB. Furthermore, with these parameters, we can develop a self-stabilization bicycle that can run straight and turn by itself. Then, by the end of the project, a research paper can be published about our journey and studies about this topic.

Project milestone: 

4th week: Have all equations of motion researched and collected

6th Week: Well-developed MATLAB models and begin prototyping physical product in SolidWorks

7th Week: Develop original literature. Create a manufacturing plan based on CAD.

9th Week: Finalize data recording. Finalize findings. Have at least a semi-functional scale model. Complete publication draft.

Team Roles:

Joaquin: Team Lead

• Facilitates team meetings, distributes tasks, and is main communicator with sponsor

Long: Software Lead

• In charge of the code for the microcontroller of the physical bicycle model

Vincent: Mechanical Lead

• Responsible for manufacturing, attachment/wiring of components to the physical model

Team Contacts:

Joaquin Garcia (joaqug1@uci.edu)

Long Do (dol4@uci.edu) 

Vincent Viratham (virathav@uci.edu)

Sponsor:

Dr. Tryphon Georgiou (tryphon@uci.edu)